I hope you can help me with this one. I try to adapt the model for a 2D simulation of a very large river and complex and deep bathymetry. In the upstream border I want to establish a flow of 7200 m3 / s, if at the upstream border I have 10 cells for example, should I divide the 7200 m3 / s between 10 and each cell assign that value as "inflow"? . Another doubt, in the water downstream, should I assign the same to value in the upstream but negative cells? Or should I leave it as an open border? If so, what would it be like to do that?
And finally, as it is a river, I understand that I must establish a value for the option "Bottom Slope - J direction from the Adjustment Options", this value corresponds to the elevation above sea level of the study area?
thank you very much in advance,
Hi Nury, for your upstream boundary you can make one boundary called “inflow” and then assign that across the 10 cells as illustrated in section 4.3.1 here: https://eemodelingsystem.atlassian.net/wiki/spaces/HOW/pages/2385726/Build+a+3D+Coastal+Model+Level+2+Step-by-Step+Guidance#Builda3DCoastalModel(Level2Step-by-StepGuidance)-TOC1 The downstream boundary can be an open boundary, in which case you need to know the water level. An example of how to set this such an open boundary is provided in the downstream BC in section 4 for this model: https://eemodelingsystem.atlassian.net/wiki/spaces/HOW/pages/2385701/Build+a+1D+River+Model+Level+1+Step-by-Step+Guidance If you have measured bathymetry data for your waterbody you should always use that rather than the fixed bottom slope option. Bathymetry is a critical aspect of your model and should be as accurate as possible.
Many thanks for replying, although I wanted to comment and ask something, I just saw and I understand that my study domain is very similar to the guide project https://eemodelingsystem.atlassian.net/wiki/spaces/HOW/pages/2385726/Build+a+3D+Coastal+Model+Level+2+Step-by-Step+Guidance#Builda3DCoastalModel(Level2Step-by-StepGuidance)-TOC1 With the difference that in my domain the border of downstream is curiously a little more upstream, ie the border of downstream is not conditioned by the tide, but still I must leave it as an open source. Can I do the same as it says in the guide referenced above? In any case, what is the “constant elevation pressure”? (image) How do I establish it for my area of study?, thank you very much, Nury Attached files 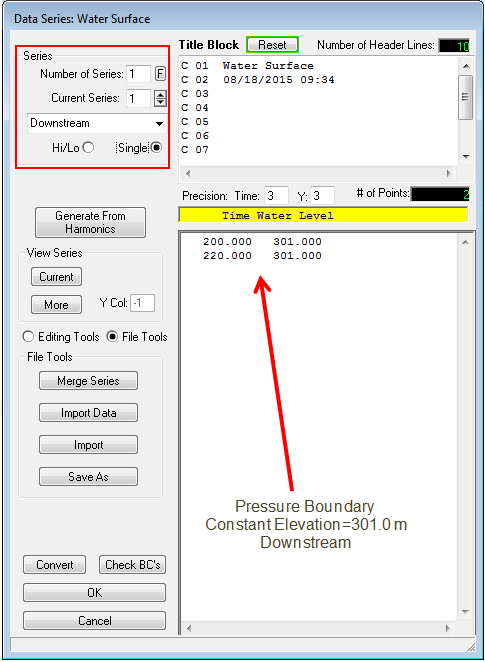
The elevation pressure is the known water level at the downstream. In the 1D River case it is constant as we are reproducing an analytical example from a text book, however in your case it should be measured data.